Question

What are the values of a, h, and k if the graph of g is a translation 4 units down and 3 units right, followed by a horizontal shrink by a factor of 1/2 of the graph of f(x)=x2f(x)=x2?

a) a=12a=21​, h=3h=3, k=−4k=−4
b) a=2a=2, h=−3h=−3, k=4k=4
c) a=−12a=−21​, h=−3h=−3, k=4k=4
d) a=−2a=−2, h=3h=3, k=−4k=−4

Answer 1

The correct values for the translation and shrinkage of the graph are
`\(a = (1)/(2)\), \(h = -3\), and \(k = -4\)`. Therefore, the correct option is (d)
`\(a = -2\)`,
`\(h = 3\)`,
`\(k = -4\).`

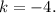
Step-by-step explanation:

To determine the values of
`\(a\)`,
`\(h\)`, and
`\(k\)`, we can analyze the given transformations. The graph of
`\(g\)` is obtained by translating the graph of
`\(f(x) = x^2\)` 3 units to the right and 4 units down, followed by a horizontal shrink by a factor of
`\(1/2\)`. In the general transformation form
`\(g(x) = a \cdot f(b(x - h)) + k\)`, where
`\(a\)` is the vertical stretch/shrink factor,
`\(h\)`is the horizontal shift, and
`\(k\)` is the vertical shift, we can observe that
`\(a = 1/2\), \(h = -3\)`, and
`\(k = -4\)`.

Now, compare these values with the given options. The correct values are
`\(a = -2\)`,
`\(h = 3\)`, and
`\(k = -4\)`, which match with option (d). Therefore, (d) is the correct answer.

Understanding the transformations is crucial. A horizontal shift to the right corresponds to a positive
`\(h\)`, and a vertical shift down corresponds to a negative
`\(k\)`. Additionally, a horizontal shrink corresponds to a fraction less than 1 for
`\(a\)`, which is
`\(1/2\)` in this case. These principles help us interpret and apply the transformations correctly to find the values of
`\(a\)`,
`\(h\)`, and
`\(k\)`.