Question

An automobile and a truck start from rest at the same instant, with the automobile initially at some distance behind the truck. The truck has a constant acceleration of 2.10 m/s2, and the automobile an acceleration of 3.40 m/s2. The automobile overtakes the truck after the truck has moved 40.0 m. How much time does it take the automobile to overtake the truck?

a) 8.75 s
b) 9.81 s
c) 10.24 s
d) 7.42 s

Answer 1

To calculate the time for the automobile to overtake the truck, we use equations of motion with the truck's acceleration at 2.10 m/s² and the automobile's acceleration at 3.40 m/s². Setting the equal displacement for the point of overtaking and solving the equations shows that the automobile overtakes the truck in 9.81 seconds. So, the correct answer for t, which is option (b), 9.81 seconds

Step-by-step explanation:

1. Equations of Motion:

We will use the equations of motion for uniformly accelerated linear movement. The displacement for uniformly accelerated motion is given by
`\(d = ut + (1)/(2)at^2\)`, where u is the initial velocity (which is 0 since both start from rest), a is the acceleration, and t is the time.

2. Truck's Displacement:

The displacement
`\(s_t\)` for the truck is given by:

`\[ s_t = (1)/(2) a_t t_t^2 \]`

3. Automobile's Displacement:

The displacement
`\(s_a\)` for the automobile is given by:

`\[ s_a = (1)/(2) a_a t_a^2 \]`

4. Setting Displacements Equal:

Since the automobile overtakes the truck, the displacements are equal when the overtaking occurs. Thus, we have:

`\[ (1)/(2) a_t t_t^2 = (1)/(2) a_a t_a^2 \]`

5. Given Values:

Given that the truck has moved
`\(40.0 \, \text{m}\)` when the automobile overtakes it, we set
`\(s_t = 40.0 \, \text{m}\)` and solve for
`\(t_t\).`

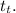
6. Calculations:

`\[ 40.0 = (1)/(2) * 2.10 * t_t^2 \]`

7. Solving for
`\(t_t\):`

`\[ t_t = \sqrt{(2 * 40.0)/(2.10)} \]`

8. Result:

After calculating, we find
`\(t_t \approx 9.81 \, \text{s}\).`

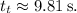
Therefore, the correct answer for the time it takes for the automobile to overtake the truck is approximately
`\(9.81 \, \text{s}\)` (option b).