Question

Find the equation of the linear function represented by the table below in slope-

intercept form.

Answer 1

f(x) = 5x - 5

Explanation:

Let the equation of the linear function is,

f(x) = mx + b

Here, m = Slope of the graph

b = y-intercept

Slope of the line passing through
`(x_1,y_1)` and
`(x_2,y_2)` is given by,

m =
`((y_2-y_1))/((x_2-x_1))`

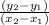
From the table attached,

Slope of the line passing through (2, 5) and (6, 25) will be,

m =
`(25-5)/(6-2)`

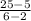
m = 5

Equation of the linear function will be,

f(x) = 5x + b

Since, a point (10, 45) lies on the function,

45 = 5(10) + b

b = 45 - 50

b = -5

Equation of the linear function will be,

f(x) = 5x - 5