Question

How to solve intercepts of linear functions

Answer 1

- X-Intercept:
`\sf (2, 0)`

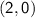
- Y-Intercept:
`\sf (0, 1)`

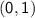
Explanation:

To find the intercepts of the linear function
`\sf x + 2y = 2`, we'll determine both the x-intercept and the y-intercept.

X-Intercept:

The x-intercept is the point where the graph intersects the x-axis, meaning that
`\sf y = 0`. To find the x-intercept, set
`\sf y = 0` and solve for
`\sf x`.

`\sf x + 2(0) = 2`

Solving for
`\sf x`:

`\sf x = 2`

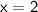
So, the x-intercept is
`\sf (2, 0)`.

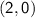Y-Intercept:

The y-intercept is the point where the graph intersects the y-axis, meaning that
`\sf x = 0`. To find the y-intercept, set
`\sf x = 0` and solve for
`\sf y`.

`\sf 0 + 2y = 2`

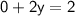
Solving for
`\sf y`:

`\sf 2y = 2 \implies y = 1`

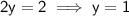
So, the y-intercept is
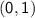`\sf (0, 1)`.