Question

What are the solutions to the system? ( , 4) and ( , )

Answer 1

The two solutions of the system of equations are (1, -4) and (-1, 54)

How to solve the system of equations?

Here we have the system of equations:

10 + y = 5x + x²

5x + y = 1

To solve it, first isolate y in the second equation:

y = 1 - 5x

Now reemplaze that in the other equation:

10 + y = 5x + x²

10 + (1 - 5x) = 5x + x²

10 + 1 - 5x - 5x - x² = 0

-x² - 10x + 11 = 0

x² + 10x - 11 = 0

Now we need to solve this quadratic equation.

`x = (-10 \pm √(10^2 - 4*1*-11) )/(2) \\\\x = (-10 \pm12 )/(2)`

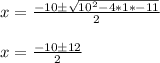
So the two solutions are:

x = (-10 + 12)/2 = 1

x = (-10 - 12)/2 = -11

Let's evaluate the linear equation to get the y-values of the solutions:

5x + y = 1

y = 1 - 5x

when x = 1

y = 1 - 5*1 = -4

when x = -11

y = 1 - 5*-11

= -1 + 55

= 54

So the solutions are:

(1, -4) and (-1, 54)