Question

Suppose that prices of a gallon of milk at various stores in one town have a mean of $3.72 with a standard deviation of $0.10. Using Chebyshev's Theorem, what is theminimum percentage of stores that sell a gallon of milk for between $3.42 and $4.02? Round your answer to one decimal place.

Answer 1

We know that the mean= 3.72$ and the sd= 0.10$

and using the Chebysev theorem we want to know whick percentage of stores will be between 3.42$ and $4.02

We can calculate the distance between the limits and the mean:

3.72 - 3.42= 0.3

k=0.3/0.1= 3

4.02-3.72= 0.3

k= 0.3/0.1 = 3

Then we are between 3 deviations from the mean

So then we can calculate the percentage required with this formula:

`1-(1)/(k^2)=1-(1)/(3^2)=1-(1)/(9)=(8)/(9)\cdot100=88.9`

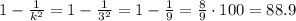
So then we expect at least 88.9%