Question

Rewrite the following equation for A and then use you result to find A when R=3

R = 4/R²+ A
Answers:
1. -23/3
2. 4/30
3. 1/3
4. -5/3

Answer 1

1. When we rewrite the equation to obtain A, the result obtained is:
`A = (4)/(R)\ -\ R^2`

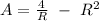
2. The value of A when R = 3, is
`-(23)/(3)` (option 1)

How to rewrite the equation and and determine A?

1. The equation,
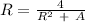`R = (4)/(R^2\ +\ A)`, can be rewritten to obtain A as illustrated below:

• Equation given from the question:
  `R = (4)/(R^2\ +\ A)`
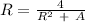
• Rewritten equation for A =?

`R = (4)/(R^2\ +\ A)\\\\Cross\ multiply\\\\R(R^2\ +\ A) = 4\\\\Divide\ both\ side\ by\ R\\\\R^2\ +\ A = (4)/(R) \\\\Subtract\ R^2\ from\ both\ side\\\\R^2\ -\ R^2 +\ A = (4)/(R)\ -\ R^2\\\\A = (4)/(R)\ -\ R^2`

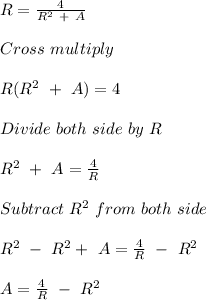
2. The value of A when R = 3, can be calculated as follow:

• Equation for
  `A = (4)/(R)\ -\ R^2`
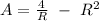
• Value of R = 3
• Value of A =?

`A = (4)/(R)\ -\ R^2\\\\A = (4)/(3)\ -\ 3^2\\\\A = (4)/(3)\ -\ 9\\\\A = (4\ -\ 27)/(3)\\\\A = -(23)/(3)`

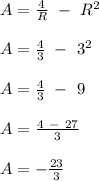
Thus, the correct answer is option 1