Question

Decide whether the following statement is true or false.Every polynomial function of degree 3 with real coefficients has exactly three real zeros.

Answer 1

Consider the next polynomial function,

`\begin{gathered} f(x)=(x+1)(x^2+1) \\ \Rightarrow f(x)=(x+1)(x-i)(x+i) \end{gathered}`

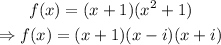
Notice that f(x) has one real zero and two complex zeros.

However, the expanded form of f(x) is

`f(x)=x^3+x^2+x+1`

Therefore, f(x) is a polynomial of degree 3 with real coefficients that has exactly 1 real zero and 2 complex zeros.

This is a counterexample of the statement. The answer is False.