Question

Fix the axis of symmetry using the formula x = -b/2a

Answer 1

We can see a quadratic function, and we have that the leading term of the function is negative. This means that the quadratic function has a maximum. We need to remember that the axis of symmetry of a quadratic function of this form is a vertical line which formula is given by:

`\begin{gathered} x=-(b)/(2a) \\ \\ \text{ For a quadratic function of the form:} \\ \\ ax^2+bx+c \end{gathered}`

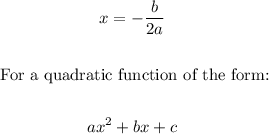
1. Then since we have that the quadratic function, in this case, is given by:

`\begin{gathered} f(x)=-9x^2+1=-9x^2+0x+1 \\ \\ \text{ Then, we have:} \\ \\ a=-9,b=0,c=1 \end{gathered}`

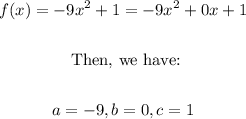
2. Now, we can apply the formula for the axis of symmetry as follows:

`\begin{gathered} x=-(b)/(2a) \\ \\ x=-(0)/(2(-9))=0 \\ \\ x=0 \end{gathered}`

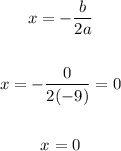
Therefore, in summary, the axis of symmetry is given by x = 0.