Answer:
32 solid balls are formed.
Explanation:
Let suppose that volume of the cone is equal to the total volume of balls, of which we derive the following formula:
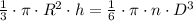 (1)
(1)
Where:
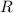 - Radius of the base of cone, measured in centimeters.
- Radius of the base of cone, measured in centimeters.
 - Height of cone, measured in centimeters.
- Height of cone, measured in centimeters.
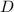 - Diameter of sphere, measured in centimeters.
- Diameter of sphere, measured in centimeters.
 - Number of balls, no unit.
- Number of balls, no unit.
Then, we clear the number of balls:
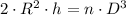

If we know that
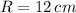 ,
,
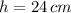 and
and
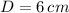 , then the number of balls is:
, then the number of balls is:
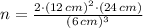

32 solid balls are formed.