Question

Hello, what is the answer please?could you please explain to me what the meaning of function and why we use it and how can we know what type of function it is .

Answer 1

A function is a mathematical relationship between inputs and outputs. It is used to describe and represent various phenomena and relationships. The type of function can be determined by analyzing the equation or graph.

Step-by-step explanation:

In mathematics, a function is a relationship between a set of inputs (called the domain) and a set of outputs (called the range) in which each input is associated with a unique output. Functions are used to describe and represent various mathematical phenomena and relationships.

Functions are used in many different areas of mathematics and other disciplines, such as physics, engineering, and computer science. They provide a way to describe how one variable depends on another, and they can be used to solve problems, make predictions, and analyze data.

To determine the type of function, you need to analyze the equation or expression that describes the relationship. Some common types of functions include linear functions, quadratic functions, exponential functions, and trigonometric functions. The type of function can be identified based on the form of the equation or the pattern of the graph.

Answer 2

ANSWERS

i) a) f(x) + 2

b) f(-x)

c) 2f(x)

ii) Exponential function

EXPLANATION

i) a) This transformation is a vertical translation 2 units up. To graph this function, we just have to copy the graph of f(x) and move it to 2 units up. Note that in the given graph, 1 unit is represented by 2 squares.

See graph in the Answers section.

b) This other transformation is a reflection over the y-axis. To graph this function, we have to draw function f(x) but flipped vertically, as if the y-axis were a mirror.

See graph in the Answers section.

c) This last transformation is a vertical stretch by a factor of 2. To graph this function, we have to take the y-values of f(x) and multiply them by 2, with the same x-value. If we do this for 3 or 4 points, and we follow the shape of the function we can graph it correctly.

See graph in the Answers section.

ii) A function is an expression that defines the relationship between two variables, one is called the independent variable and the other is the dependent variable. We use functions to show how the dependent variable changes when the independent variable changes. For example, if we want to show how fast a person is moving, we would have a function where the independent variable is time and the dependent variable is the person's position.

This function is an exponential function. It has all the characteristics for the domain graphed:

• The range is y > 0

,

• There is a horizontal asymptote at the x-axis when x approaches negative infinity

,

• The graph increases to infinity when x approaches positive infinity

,

• The graph is continuous and smooth

Also, when x = 0, f(0) = 1, so the function most likely represents the equation,

`f(x)=a^(bx)`

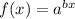
Where a and b are real numbers.