Question

A 4kg object is moving at 5 m/s . A 10-N force is applied in the direction of motion and if the object has a final velocity 10m /s. calculate the distance that the object moves

Answer 1

Distance, S = 15m

Step-by-step explanation:

Given the following data;

Mass = 4kg

Force = 10N

Initial velocity = 5m/s

Final velocity = 10m/s

To find the distance;

First of all, we would calculate the acceleration

Force = mass * acceleration

10 = 4*acceleration

Acceleration = 10/4

Acceleration = 2.5m/s²

Now to find the distance, we would use the third equation of motion.

`V^(2) = U^(2) + 2aS`

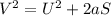
Where;

• V represents the final velocity measured in meter per seconds.
• U represents the initial velocity measured in meter per seconds.
• a represents acceleration measured in meters per seconds square.

S represents the displacement measured in meters.

Substituting into the equation, we have;

10² = 5² + 2*2.5*S

100 = 25 + 5S

5S = 100 - 25

5S = 75

Distance, S = 75/5

Distance, S = 15m