Question

Write a quadratic equation in factored form if it has x-intercepts 2 and -1 and y-intercept 6.

Answer 1

We have to write a quadratic equation, in factored form, that has an x-intercept at x=2 and x=-1, and a y-intercept 6.

As the x-intercepts are the roots of the function, we can write the equation as:

`y=a(x-2)(x+1)`

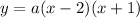
The parameter a will be defined in order to have a y-intercept at y=6. That means that, when x is 0, the value of the function is y=6.

Then, we can replace x with 0 and y with 6 and find the value of a:

`\begin{gathered} y=a(x-2)(x+1) \\ 6=a(0-2)(0+1) \\ 6=a(-2)(1) \\ 6=-2a \\ a=(6)/(-2) \\ a=-3 \end{gathered}`

With the value of a=-3, we can write the factorized form of the equation as:

`y=-3(x-2)(x+1)`

Graph:

Answer: y=-3(x-2)(x+1)