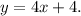Step-by-step explanation
Let's recall that the slope-intercept equation of a line has the form
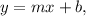
where "m" is the slope, and "b" is the y-intercept. We know that the y-intercept is 4; then, our equation becomes
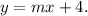
The trick to finding the slope is to note that the point (1,8) lies on the line; namely, we can evaluate the equation of the line in this point:
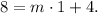
Solving this equation for m, we get
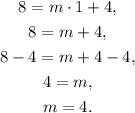
Answer
The equation of the line satisfying the provided conditions is