Answer:
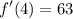
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Distributive Property
Algebra I
- Expand by FOIL (First Outside Inside Last)
- Factoring
- Function Notation
- Terms/Coefficients
Calculus
Derivatives
The definition of a derivative is the slope of the tangent line.
Limit Definition of a Derivative:
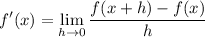
Explanation:
Step 1: Define
f(x) = 7x² + 7x + 3
Slope of tangent line at x = 4
Step 2: Differentiate
- Substitute in function [Limit Definition of a Derivative]:
![\displaystyle f'(x)= \lim_(h \to 0) ([7(x + h)^2 + 7(x + h) + 3]-(7x^2 + 7x + 3))/(h)](https://img.qammunity.org/2022/formulas/mathematics/high-school/33mdbzul76nvxawg2suijr9pt4817d5yjy.png)
- [Limit - Fraction] Expand [FOIL]:
![\displaystyle f'(x)= \lim_(h \to 0) ([7(x^2 + 2xh + h^2) + 7(x + h) + 3]-(7x^2 + 7x + 3))/(h)](https://img.qammunity.org/2022/formulas/mathematics/high-school/doehniux5pps3n8s8ssr6w9aklhq90ewug.png)
- [Limit - Fraction] Distribute:
![\displaystyle f'(x)= \lim_(h \to 0) ([7x^2 + 14xh + 7h^2 + 7x + 7h + 3] - 7x^2 - 7x - 3)/(h)](https://img.qammunity.org/2022/formulas/mathematics/high-school/sc4s3syxgskwtlhsge713jvia3d46d0gay.png)
- [Limit - Fraction] Combine like terms (x²):
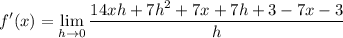
- [Limit - Fraction] Combine like terms (x):
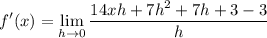
- [Limit - Fraction] Combine like terms:
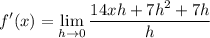
- [Limit - Fraction] Factor:
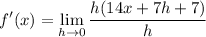
- [Limit - Fraction] Simplify:
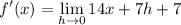
- [Limit] Evaluate:
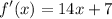
Step 3: Find Slope
- Substitute in x:
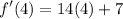
- Multiply:
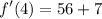
- Add:
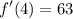
This means that the slope of the tangent line at x = 4 is equal to 63.
Hope this helps!
Topic: Calculus AB/1
Unit: Chapter 2 - Definition of a Derivative
(College Calculus 10e)