Solving the Probability and Statistics Problems for Chronic Sinusitis
a) Probability of exactly 3 with chronic sinusitis:
Using the binomial probability formula:
P(k successes in n trials) =
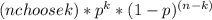
Plugging in the values:
P(3 successes in 17 trials) = (17 choose 3) *
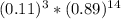
≈ 0.0133
b) Probability of fewer than 4 with chronic sinusitis:
This involves calculating the probabilities of 0, 1, 2, and 3 successes and adding them up:
P(fewer than 4 successes) = P(0 successes) + P(1 success) + P(2 successes) + P(3 successes)
Using the binomial formula for each probability and adding them up:
P(fewer than 4 successes) ≈ 0.0036 + 0.0358 + 0.1379 + 0.1729 ≈ 0.3502
c) Probability of more than 4 with chronic sinusitis:
This is the complement of the previous probability:
P(more than 4 successes) = 1 - P(fewer than 4 successes) ≈ 1 - 0.3502 ≈ 0.6498
d) Probability of at most 3 with chronic sinusitis:
This includes the probabilities of 0, 1, 2, and 3 successes:
P(at most 3 successes) = P(0 successes) + P(1 success) + P(2 successes) + P(3 successes)
≈ 0.0036 + 0.0358 + 0.1379 + 0.1729 ≈ 0.3502
e) Mean number of Americans with chronic sinusitis:
This is the expected value, calculated as:
Mean = n * p = 17 * 0.11 ≈ 1.87
Therefore, on average, we expect 1.87 Americans in the sample to suffer from chronic sinusitis.
f) Standard deviation of the number of Americans with chronic sinusitis:
This is calculated using the formula:
Standard deviation = √(n * p * (1-p)) = √(17 * 0.11 * 0.89) ≈ 1.2901
Therefore, the standard deviation is approximately 1.2901, indicating the typical spread in the number of Americans with chronic sinusitis in such samples.
Complete Question:
The Centers for Disease Control and Prevention (CDC) estimates that 11% of American adults suffer from chronic sinusitis (inflammation of the sinus). A random sample of 17 American are selected. Round answers to at least 4 decimal places.
a) Compute the probability that exactly 3 in the sample suffer from chronic sinusitis.
b) Compute the probability that there are fewer than 4 in the sample that suffer from chronic sinusitis.
c) Compute the probability that there are more than 4 in the sample that suffer from chronic sinusitis.
d) Compute the probability that there are at most 3 in the sample that suffer from chronic sinusitis.
e) Compute the mean number of Americans that suffer from chronic sinusitis.
f) Compute the standard deviation of the number of Americans that suffer from chronic sinusitis.