Question

There are currently 400,000 cats in the San Diego area. The number of cats in San Diego increases each year by 2.5 % A) how many cats will there be in the year 2036 ? B) how long will it be before the number of cats doubles ?

Answer 1

a) 436529 cats b) Approximately 278 years

1) Gathering the data

400,000 cats

Increases yearly by 2.5%

2) Let's write that growth as a function. Note that we must rewrite 2.5% as purely decimal 0.0025. A growth of 2.5 must be written as 1.0025.

Because every time we multiply by 1.0025 we are multiplying the number and 2.5%. Considering that there are currently, in this 1st year 400,000 cats 2036 then this will be 35 years after

`\begin{gathered} y=400000(1.0025)^n \\ y=400000(1.0025)^(35) \\ y=436529.23\text{ }\cong436,529\text{ } \end{gathered}`

So considering we're in the first year, 35 years after in 2036 there'll be 436,529

b) Since n= is the number of years in that function, and y stands for the number of cats.

`\begin{gathered} 800,000=400,000(1.0025)^n \\ (800,000)/(400,00)=(400,000)/(400,000)(1.0025)^n \\ 2=(1.0025)^n \\ \log 2\text{ =}\log (1.0025)^n \\ 0.3=^{}n1.08\cdot10^(-3) \\ n=(0.3)/(1.08\cdot10^(-3)) \\ n=277.8 \\ \end{gathered}`

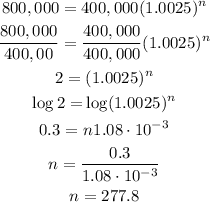
So, it will take at this rate approximately 278 years for the population of cats doubles.