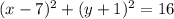Answer:
The equation of the circle is;
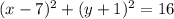
Step-by-step explanation:
Given that the circle has a diameter with endpoints located at (7,3) and (7,-5).
The diameter of the circle is the distance between the two points;
![\begin{gathered} d=\sqrt[]{(7-7)^2+(3--5)^2_{}} \\ d=\sqrt[]{(0)^2+(3+5)^2_{}} \\ d=\sqrt[]{64} \\ d=8 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/8xk7wfjdgmpmlw4s8n3t.png)
The radius of the circle is;
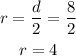
The center of the circle is at the midpoint of the line of the diameter.
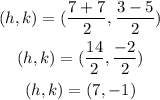
Applying the equation of a circle;

Substituting the given values;
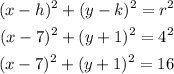
Therefore, the equation of the circle is;