Final Answer:
Apply the power rule for integration to each term:
 . This yields
. This yields
 , matching option (a).
, matching option (a).
Step-by-step explanation:
The given indefinite integral
 dx can be solved by applying the power rule for integration, which states that
dx can be solved by applying the power rule for integration, which states that
 . Applying this rule to each term individually, the integral becomes
. Applying this rule to each term individually, the integral becomes
 , where C is the constant of integration. In the first term,
, where C is the constant of integration. In the first term,
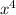 integrates to
integrates to
 , in the second term,
, in the second term,
 integrates to
integrates to
 , and in the third term, the integral of the constant term 5 is 5x. The constant of integration, denoted by C, represents an arbitrary constant that can take any real value.
, and in the third term, the integral of the constant term 5 is 5x. The constant of integration, denoted by C, represents an arbitrary constant that can take any real value.
Option (a)
 perfectly aligns with the derived result, confirming it as the correct answer. The power rule is a fundamental technique in calculus, establishing a direct relationship between derivatives and integrals for power functions.
perfectly aligns with the derived result, confirming it as the correct answer. The power rule is a fundamental technique in calculus, establishing a direct relationship between derivatives and integrals for power functions.
Understanding and applying such rules are crucial for solving a wide range of integration problems efficiently, providing a systematic approach to finding antiderivatives.