Answer: (3,2)
Step-by-step explanation: Diagonal is a line connecting two opposite points of a form. In parallelograms, there two diagonals and they bisect each other, i.e., the point where the diagonals meet divide them into two segments with the same measure. In short, diagonals meet at their midpoint.
The way to determine midpoint is given by
(x,y) =
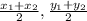
(x,y) =

(x,y) = (3,2)
The coordinates where diagonals of parallelogram GHJK intersect are (3,2).