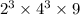Question

Simplify completely. Leave your answer in exponential form:

Answer 1

`{2}^(3) * {4}^(3) * 9`

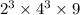
Explanation:

Using the division rule when dealing with exponents

`\frac{b {}^(h) }{b {}^(j) } = b {}^(h - j)`

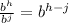
meaning that when we divide exponents that have the same base but different powers we can subtract the power of the bottom exponent from the top exponet.

so using that we are going to do 2, 4 and 9

`\frac{2 {}^(8) }{ {2}^(5) } = {2}^{8 - 5 = 3 \: \: \: \: \: 2 {}^(3) }`

`\frac{ {4}^(4) }{ {4}^(1) } = {4}^(4 - 1) \: \: \: {4}^(3)`

`\frac{9 {}^(9) }{ {9}^(8) } = {9}^(9 - 8) = 9 {}^(1)`

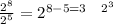
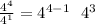
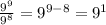
Combine all the terms and we get

`{2}^(3) * 4 {}^(3) * {9}`