Question

Rupees 8250 is divided among three friends A B and C such that ratio of their share is 6:7:9 the share of each

Answer 1

sum of ratios : 22

part 1 : 6/22*8250 = 2250

part 2 : 7/22*8250 = 2625

part 3 : 9/22*8250 = 3375

Explanation:

Answer 2

Shares of A = Rs 2250

Shares of B = Rs 2625

Shares of C = Rs 3375

Explanation:

Let the shares of A, B, and C be
`\sf 6x`,
`\sf 7x`, and
`\sf 9x` respectively, where
`\sf x` is a constant.

The total sum of the shares is given as
`\sf 6x + 7x + 9x = 22x`, and this is equal to Rs. 8250.

So, we can set up an equation and solve for
`\sf x`:

`\sf 22x = 8250`

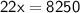
Now, solve for
`\sf x`:

`\sf x = (8250)/(22)`

`\sf x = 375`

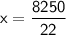
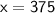
Now that we have the value of
`\sf x`, we can find the individual shares:

- Share of A (
`\sf 6x`):
`\sf 6 * 375 = 2250` rupees

- Share of B (
`\sf 7x`):
`\sf 7 * 375 = 2625` rupees

- Share of C (
`\sf 9x`):
`\sf 9 * 375 = 3375` rupees

So, the shares of A, B, and C are 2250 rupees, 2625 rupees, and 3375 rupees respectively.