Question

10. Write a polynomial in factored form with a single root at 0 and a double

root at 3 that decreases as x approaches infinity.

Answer 1

One possible polynomial is given by:
`f(x) = -x(x-3)^2`

Explanation:

Zeros of a function:

Given a polynomial f(x), this polynomial has roots
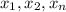`x_(1), x_(2), x_(n)` such that it can be written as:
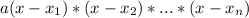`a(x - x_(1))*(x - x_(2))*...*(x-x_n)`, in which a is the leading coefficient.

Single root at 0, double root at 3:

This means that:

`f(x) = a(x - 0)(x - 3)(x - 3) = ax(x-3)^2`

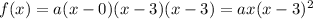
Decreases as x approaches infinity.

This means that the leading coefficient should be negative. I am going to use -1. So

`f(x) = -x(x-3)^2`