Question

A block of metal has a volume of 13.2 inches cubed and weighs 5.22 pounds. What is the density in grams per cubic meter?

Answer 1

The density of the block of metal is approximately 318.18 grams per cubic meter.

Step-by-step explanation:

Density is defined as the mass of an object per unit volume. To calculate density, we use the formula:

`\[Density (ρ) = (Mass (m))/(Volume (V))\]`

Given that the volume of the metal block is
`\(13.2 \, \text{in}^3\) and the weight is \(5.22 \, \text{pounds}\)`, we first need to convert these units to a consistent system. Converting the volume from cubic inches to cubic meters, we use the conversion factor
`\(1 \, \text{in}^3 = 0.000016387 \, \text{m}^3\):`

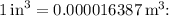
`\[Volume (V) = 13.2 \, \text{in}^3 * 0.000016387 \, \text{m}^3/\text{in}^3\]`

Next, we convert the weight from pounds to grams, using the conversion factor
`\(1 \, \text{pound} = 453.592 \, \text{grams}\):`

`\[Mass (m) = 5.22 \, \text{pounds} * 453.592 \, \text{grams/pound}\]`

Now, we substitute these values into the density formula:

`\[Density (ρ) = \frac{5.22 \, \text{pounds} * 453.592 \, \text{grams/pound}}{13.2 \, \text{in}^3 * 0.000016387 \, \text{m}^3/\text{in}^3}\]`

After performing the calculations, the density is approximately
`\(318.18 \, \text{grams/m}^3\)`. Thus, the density of the metal block is approximately
`\(318.18 \, \text{grams per cubic meter}\)`, indicating the mass of 318.18 grams occupies each cubic meter of space.