Question

If the graph of f(x) = 3^x is reflected over the x-axis, what is the equation of the new graph?

A. g(x) = -(3)^x
B. g(x) = (1/3)^x
C. G(x) = - (1/3)^x
D. G(x) = 3^-x

Answer 1

`\[ \text{If the graph of } f(x) = 3^x \text`{ is reflected over the x-axis, the equation of the new graph is }
`\boxed{\text{C. } G(x) = - (1/3)^x}. \]` opiton.c

Step-by-step explanation:

When a function is reflected over the x-axis, each y-coordinate of the points on the original graph becomes its opposite. In the case of
`\(f(x) = 3^x\),` reflecting over the x-axis transforms it into
`\(g(x) = -3^x\).`However, the options are in terms of the base, so we need to express
`\(g(x)\)` using the base
`\(1/3\).`

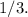
Recall that
`\(3^(-x) = (1)/(3^x)\).` So, to obtain the equivalent expression with a negative sign, we get
`\(g(x) = -3^x = -(1)/(3^(-x))\)`. Therefore, the correct answer is
`\(G(x) = - (1/3)^x\),` corresponding to option C.

In summary, reflecting a function over the x-axis negates the original function, and in this case, expressing it in terms of the base
`\(1/3\)` gives us the correct transformed function. Thus, option C,
`\(G(x) = - (1/3)^x\),` is the accurate equation for the reflected graph of
`\(f(x) = 3^x\).`