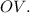Final Answer:
Given angle V below, you can conclude that angle OS is congruent to

Step-by-step explanation:
In the provided diagram, let
 be denoted as
be denoted as
 The congruence of corresponding angles in a geometric figure implies the congruence of the associated line segments. Therefore,
The congruence of corresponding angles in a geometric figure implies the congruence of the associated line segments. Therefore,
 is congruent to
is congruent to

Next, consider the given options. Option D,
 , corresponds to
, corresponds to
 in the figure. By the Corresponding Angles Postulate,
in the figure. By the Corresponding Angles Postulate,
 is congruent to
is congruent to
 Thus, the correct conclusion is that
Thus, the correct conclusion is that
 is congruent to
is congruent to
 and the answer is D.
and the answer is D.
To further validate this, one can use the definition of congruent angles. Two angles are congruent if and only if they have the same measure. In this case
 and
and
 share the same measure, confirming the congruence. In mathematical terms, this can be expressed as
share the same measure, confirming the congruence. In mathematical terms, this can be expressed as
 due to the Corresponding Angles Postulate. Therefore, the correct answer is option D,
due to the Corresponding Angles Postulate. Therefore, the correct answer is option D,