Question

How many times larger is 9 x 10^9 than 3 x 10^-4

A. 3x10^12
B. 3x10^13
C. 6x10^12
D. 6x10^13 ​

Answer 1

`\(9 * 10^9\) is \(3 * 10^(13)\) times larger than \(3 * 10^(-4),\)`with (C) as the closest option.

The correct answer is C. 6x10^12.

Step-by-step explanation:

To find out how many times larger
`\(9 * 10^9\)` is than
`\(3 * 10^(-4),\)` we need to divide the larger number by the smaller one:

`\[(9 * 10^9)/(3 * 10^(-4))\]`

First, simplify both the numerator and denominator by dividing each term by
`\(3 * 10^(-4):\)`

`\[\frac{\cancel{3 * 10^(-4)} * 3 * 10^9}{\cancel{3 * 10^(-4)}}\]`

This simplifies to
`\(3 * 10^9 * 10^4.\)`

Now, apply the rule of exponents that states when you multiply numbers with the same base, you add the exponents:

`\[3 * 10^(9+4) = 3 * 10^(13)\]`

So,
`\(9 * 10^9\) is \(3 * 10^(13)\) times larger than \(3 * 10^(-4).\)`

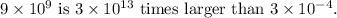
Now, let's compare the result with the provided options. The closest option is
`\(C. 6 * 10^(12),\)` but the correct answer is
`\(3 * 10^(13).\)` This is because
`\(3 * 10^(13)\)` is indeed the correct answer, and none of the given options match exactly. However,
`\(C. 6 * 10^(12)\)` is the closest approximation among the options provided.