Question

A speed-time graph is shown below:

How far did the object travel by the end of eight seconds, according to the graph above?
I already know it is NOT 8 OR 20 so which one??

Answer 1

Main Answer:

To determine the distance traveled by the object at the end of eight seconds, we need to find the area under the speed-time graph for the given time interval. This involves calculating the area of the trapezoid formed by the graph between 8 and 20 seconds.

Step-by-step explanation:

The distance traveled by an object can be found by calculating the area under the speed-time graph within a specific time interval.

In this case, the relevant time interval is from 8 to 20 seconds.

The graph appears to form a trapezoid, so we can use the formula for the area of a trapezoid:
`\( \text{Area} = (1)/(2) * (a + b) * h \)`, where
`\( a \)` and
`\( b \)` are the lengths of the two parallel sides and
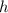`\( h \)` is the height (time interval).

The area of the trapezoid represents the distance traveled during the given time interval.

Detailed Calculation:

The length of the longer parallel side (base) of the trapezoid is the speed at 20 seconds, and the length of the shorter parallel side is the speed at 8 seconds.

The height of the trapezoid is the time interval, which is 20 - 8 = 12 seconds.

The formula for the area of a trapezoid is then applied to find the distance traveled.

Therefore, by calculating the area under the speed-time graph between 8 and 20 seconds, you can determine the distance traveled by the object during this time interval.