Question

Ren sets aside $1,000 into an online savings account with an annual

interest rate of 2.3%, compounded annually. How long will it take for the


money in his account to double?

Answer 1

It will take 30.48 for the money in his account to double.

Explanation:

Interest compounded anually:

With an investment of P, the amount compounded annualy after t years that you will have is given by:

`A(t) = P(1+r)^(t)`

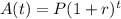
In which r is the interest rate, as a decimal.

Ren sets aside $1,000 into an online savings account with an annual interest rate of 2.3%

This means that
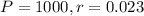`P = 1000, r = 0.023`. So

`A(t) = P(1+r)^(t)`

`A(t) = 1000(1+0.023)^(t)`

`A(t) = 1000(1.023)^(t)`

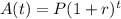
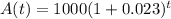
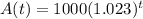
How long will it take for the money in his account to double?

This is t for which A(t) = 1000*2 = 2000. So

`A(t) = 1000(1.023)^(t)`

`2000 = 1000(1.023)^(t)`

`(1.023)^(t) = (2000)/(1000)`

`(1.023)^(t) = 2`

`\log{(1.023)^(t)} = \log{2}`

`t\log{1.023} = \log{2}`

`t = \frac{\log{2}}{\log{1.023}}`

`t = 30.48`

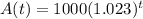
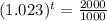
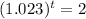
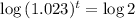
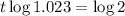
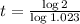
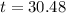
It will take 30.48 for the money in his account to double.