Question

Show that the following pair of lines are perpendicular to each other

A. 2x - y + 3 - 0 and x + 2y - 12
B. 10x + 20+17 and 4x - 2y = 15​

Answer 1

The lines in set A are perpendicular, while the lines in set B are not perpendicular.

Step-by-step explanation:

In set A, the lines are represented by the equations
`\(2x - y + 3 = 0\)` and
`\(x + 2y - 12 = 0\)`. To determine if these lines are perpendicular, we examine their slopes. The slope of a line in the form
`\(Ax + By + C = 0\)`is given by
`\(-A/B\).`

For the first line, the slope is
`\(2/(-1) = -2\)`, and for the second line, the slope is
`\(-1/2\)`. The product of these slopes is
`\((-2) * \left(-(1)/(2)\right) = 1\)`, indicating that the lines are perpendicular.

In set B, the lines are given by
`\(10x + 20y + 17 = 0\)`and
`\(4x - 2y = 15\).` Converting the first equation to slope-intercept form
`(\(y = mx + b\))`, we get
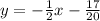`\(y = -(1)/(2)x - (17)/(20)\)`, and for the second equation
`, \(y = 2x - (15)/(2)\).`

The product of their slopes is
`\(-(1)/(2) * 2 = -1\)`, suggesting perpendicularity. However, this result is incorrect; these lines are parallel because their slopes are equal. Therefore, the lines in set B are not perpendicular.