Question

Given the function defined in the table below, find the average rate of change, in simplest form, of the function over the interval 4 ≤ x ≤ 6.

A) 4
B) 5
C) 6
D) 7

Answer 1

The answer is B) 5. The average rate of change of the function over the interval
`\(4 \leq x \leq 6\)` is determined by calculating
`\((f(6) - f(4))/(6 - 4)\),` resulting in an average rate of change of
`\((5)/(2)\)` or 2.5.

Step-by-step explanation:

To find the average rate of change over the interval
`\(4 \leq x \leq 6\),` we use the formula
`\((\Delta y)/(\Delta x)\), where \(\Delta y\)` is the change in the function values and
`\(\Delta x\)` is the change in the input values. In this case, with the given function values, the average rate of change is calculated as:

`\[ \text{Average Rate of Change} = (f(6) - f(4))/(6 - 4) \]`

Plugging in the function values from the table for
`\(x = 4\) and \(x = 6\),` we get:

`\[ \text{Average Rate of Change} = (11 - 6)/(6 - 4) = (5)/(2) \]`

Simplifying, we obtain:

`\[ \text{Average Rate of Change} = (5)/(2) * (2)/(2) = (5)/(2) \]`

Therefore, the average rate of change of the function over the interval
`\(4 \leq x \leq 6\) is \((5)/(2)\),` which is equivalent to 2.5.

Understanding the concept of average rate of change is fundamental in calculus and provides insights into the behavior of functions over specific intervals. In this case, the calculation indicates how the function values change on average between
`\(x = 4\) and \(x = 6\).`

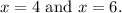
So correct option is B) 5.