Final Answer:
a.
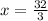
b.
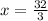
c.
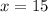
Step-by-step explanation:
For equation a,
 adding 10 to both sides gives
adding 10 to both sides gives
 Dividing by 9, we have
Dividing by 9, we have
 . Solving for
. Solving for
 or
or
 results in
results in
 or
or
 but only
but only
 is valid.
is valid.
For equation b,
 subtracting 4 yields
subtracting 4 yields
 Dividing by 3 gives
Dividing by 3 gives
 . Solving for
. Solving for
 or
or
 gives x = 7 or x = -9, but only x = 4 satisfies the original equation.
gives x = 7 or x = -9, but only x = 4 satisfies the original equation.
Lastly, for equation c,
 , subtracting 7 gives
, subtracting 7 gives
 Dividing by 4 results in
Dividing by 4 results in
 Solving for
Solving for
 or
or
 gives x = 24 or x = -6, but only x = 15 satisfies the original equation. In conclusion, the correct solutions for the given equations are
gives x = 24 or x = -6, but only x = 15 satisfies the original equation. In conclusion, the correct solutions for the given equations are
 for a, x = 4 for b, and x = 15 for c.
for a, x = 4 for b, and x = 15 for c.