Question

1. Ingrid will start college next year. She wasapproved for 10-year unsubsidized Federal Loanfor the amount of $15,000 at 4.29%a) How much interest will Ingrid accrue for 4.5 yearsnonpayment period?b) What will the new principal be when she beginsmaking loan payments?c) How much interest will she pay over the life of theloan?2. Suppose Ingrid only paid the interest during her 4years of school and the six-month grace period.What will she now pay in interest over the term ofthe loan?3. Ingrid made her last monthly interest only paymenton September 1 Her next payment is due onOctober 1. What will be the amount of interestonly payment?4. Suppose Ingrid has decided to apply for a privateloan rather then a federal loan. She has beenapproved for a 10 year loan with APR of 7.8%a) What is her monthly payments?b) What is the total amount she will pay back?c) What is total interest amount?

Answer 1

Given:

`\begin{gathered} Principal=15,000 \\ rate(r)=4.9\%=0.049 \\ time(t)=10years \end{gathered}`

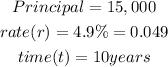
To Determine: (a) How much interest will Ingrid accrue for 4.5 years non payment period

Solution

Calculate the amount accrued for 4.5years

The formula for finding amount for compound interest is

`A=P(1+r)^(nt)`

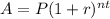
Substitute the given into the formula

`\begin{gathered} A=15000(1+0.049)^(4.5) \\ A=15000(1.049)^(4.5) \\ A=18602.91 \end{gathered}`

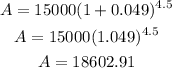
Step 2: Calculate the interest accrued for 4.5 years

`\begin{gathered} I=A-P \\ I=18602.91-15000 \\ I=3602.91 \end{gathered}`

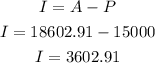
(a) Hence the interest Ingrid will accrued for 4.5 years non-payment period is $3,602.91

(b) The new principal when she begins making loan payments will be the amount accrued for 4.5years nonpayment period. This is as calculated above, which is

$18,602.91

(c) To Determine how much interest will she pay over the life of the loan

Note that the life of the loan is 10 years

`So,t=10`

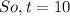
Substitute the given into the formula for finding the amount as shown below

`\begin{gathered} A=15000(1+0.049)^(10) \\ A=15000(1.049)^(10) \\ A=24201.71 \end{gathered}`

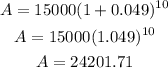
Use the amount to calculate the interest of the life of the loan

`\begin{gathered} I=A-P \\ I=24201.71-15000 \\ I=9201.71 \end{gathered}`

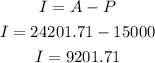
Hence, the interest she would pay over the life of the loan is $9,201.71