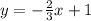Answer (assuming it is allowed to be written in slope-intercept form):
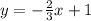
Explanation:
When knowing a line's y-intercept and its slope, you can write its equation using slope-intercept form, or
 .
.
1) First, find the slope of the line. Read the graph and look at the two points marked on the line - they are (0,1) and (3,-1). Use the slope formula
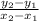 , substitute the x and y values of those points, and solve:
, substitute the x and y values of those points, and solve:
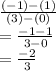
Thus, the line's slope is
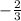 .
.
2) Now, use the slope-intercept formula,
 , to write an equation. Substitute the
, to write an equation. Substitute the
 and
and
 for real values.
for real values.
The
 represents the slope, so substitute
represents the slope, so substitute
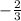 in its place. The
in its place. The
 represents the y-value of the y-intercept, or the point at which the line crosses the y-axis. Looking at the graph, we can see that the line intersects the y-axis at (0,1). Thus, substitute 1 for
represents the y-value of the y-intercept, or the point at which the line crosses the y-axis. Looking at the graph, we can see that the line intersects the y-axis at (0,1). Thus, substitute 1 for
 . This gives the following equation and answer:
. This gives the following equation and answer: