Question

What is an equation of the line that passes through the points (-6, 2) and

(-4, -1)? Put your answer in fully reduced form.

Answer 1

Answer (assuming the equation can be written in point-slope form):

`y-2 = -(3)/(2)(x+6)`

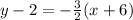
Step-by-step explanation:

When knowing a point the line crosses through and its slope, you can write an equation in point-slope form, or
`y-y_1= m (x-x_1)`.

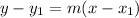1) First, find the slope of the line. Use the slope formula
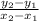`(y_2-y_1)/(x_2-x_1)` and the x and y values of the two points given, then solve like so:

`((-1)-(2))/((-4)-(-6))\\= (-1-2)/(-4+6)\\= (-3)/(2)`

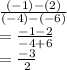
Thus, the slope is
`-(3)/(2)`.

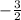2) Now, use point-slope form,
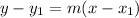`y-y_1= m (x-x_1)`. Substitute the
`m`,
`x_1`, and
`y_1` for real values.

The
`m` represents the slope, so substitute
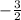`-(3)/(2)` in its place. The
`x_1` and
`y_1` represent the x and y values of one point the line crosses through. Any of the two points will work, and I chose (-6,2) for this answer. So, substitute -6 for

`y-(2)= -(3)/(2)(x-(-6)\\y-2 = -(3)/(2)(x+6)`