Question

During a hockey game, two hockey players (m1 = 82kg and m2 = 76kg) collide head on in a 1 dimensional perfectly instant collision. If the first hockey player is moving to the left at a velocity 4.2 m/s and the second hockey player is moving in the opposite direction at a velocity of 3.4 m/s, how fast are they both moving after they collide, assuming they stick together after they collide? How much kinetic energy is lost as a result of the collision?

Answer 1

Given:

The mass of player 1 is m1 = 82 kg

The initial velocity of player 1 is

`v_(i1)=\text{ 4.2 m/s}`

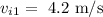
towards left.

The mass of player is m2 = 76 kg

The initial velocity of player 2 is

`v_(i2)=\text{ -3.4 m/s}`

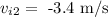
in opposite direction.

Required:

The final velocity after the collision assuming they stick together.

The loss of kinetic energy after collision.

Step-by-step explanation:

According to the conservation of momentum, the final velocity will be

`\begin{gathered} m1v_(i1)+m2v_(i2)=(m1+m2)v_f \\ v_f=(m1v_(i1)+m2v_(i2))/(m1+m2) \\ =\text{ }((82*4.2)-(76*3.4))/(82+76) \\ =0.544\text{ m/s} \end{gathered}`

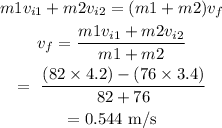
The loss in kinetic energy will be

`\begin{gathered} \Delta KE=KE_(after)-KE_(before) \\ =(1)/(2)(m1+m2)(v_f)^2-(1)/(2)m1(v_(i1))^2-(1)/(2)m2(v_(i2))^2 \\ =(1)/(2)(82+76)(0.544)^2-(1)/(2)*82*(4.2)^2-(1)/(2)*76*(3.4)^2 \\ =23.38-723.24-439.28 \\ =-1139.14\text{ J} \end{gathered}`

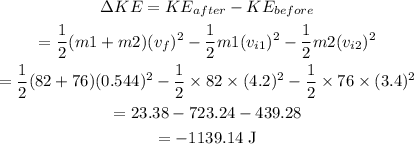
Final Answer:

The final velocity after the collision assuming they stick together is 0.544 m/s.

The loss of kinetic energy after the collision is 1139.14 J.