The equation of the line is y≈3.3x+7.
The slope of the line is equal to the tangent of the angle it makes with the positive x-axis.
Therefore, the slope of the line is equal to tan(74.78 ∘ )≈3.3.
The y-intercept of the line is 7, since the line passes through the point (0,7).
Therefore, the equation of the line is y≈3.3x+7.
Here are the steps to solve for the equation of the line:
Recall the formula for the slope of a line: m=
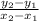
, where (
 ,
,
 ) and (
) and (
 ,
,
 ) are two points on the line.
) are two points on the line.
Use the given angle to find the slope: Since the line makes a 74.78-degree angle with the positive x-axis, the slope is equal to
tan(74.78∘ )≈3.3.
Use the given point to find the y-intercept: Since the line passes through the point (0,7), the y-intercept is 7.
Substitute the slope and y-intercept into the equation of the line: y=mx+b, where m is the slope and b is the y-intercept. In this case, the equation is y≈3.3x+7.
Therefore, the equation of the line is y≈3.3x+7.