Question

The points (3, -6) and (6, r) lie on a line with slope -2. Find the missing coordinate r

Answer 1

Explanation:

The slope = (y1-y1) / (x2-x1) where the 2 points are (x1, y1) and (x2, y2).

So here we have:

(r - (-6) / (6-3) = -2

(r + 6) / 3 = -2

r + 6 = -2*3

r + 6 = -6

r = -6 - 6

r = -12.

Answer 2

r = - 12

Explanation:

calculate the slope between the 2 given points , then equate to - 2

calculate the slope m using the slope formula

m =
`(y_(2)-y_(1) )/(x_(2)-x_(1) )`

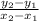
let (x₁, y₁ ) = (3, - 6 ) and (x₂, y₂ ) = (6, r )

substitute these values into the formula for m

m =
`(r-(-6))/(6-3)` =
`(r+6)/(3)`

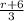
now, equate the expression for m to - 2

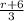`(r+6)/(3)` = - 2 ( multiply both sides by 3 )

r + 6 = - 6 ( subtract 6 from both sides )

r = - 12