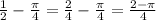Question

What is the exact value of sin(cos^−1(−√3/2))+tan^−1(sin(−π/2))?

Answer 1

`(2-\pi)/(4)`

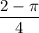
Explanation:

we are given the expression

`\sin( \cos^(−1)(− √(3)/2))+ \tan^(−1)( \sin(−π/2))`

to find the value of

`\sin( \cos^( - 1) (x) )`

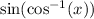
we consider an angle p such that

`p = \cos^( - 1) (x)`

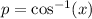
next, we have

`\sin(p) = \sqrt{1 - \cos^(2)( p)}`

and substituting back the value of p, we have

`\sqrt{1-\cos^2(\cos^(-1)x)} = √(1-x^2)\\\implies \sin(\cos^(-1)x)=√(1-x^2)`

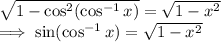
so, letting x = - sqrt(3)/2,

`(-(√(3))/(2))^2=(3)/(4)`

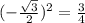
and then, we have

`\sqrt{1-(3)/(4)}=\sqrt{(1)/(4)}=(1)/(2)`

`\therefore \sin(\cos^(-1)(-(√(3))/(2)))=(1)/(2)`

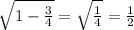
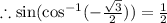
our next part is on

`\tan^(-1)(\sin (-(\pi)/(2)))`

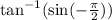
this is easier, as we know that

`\sin(-(\pi)/(2))=-\sin((\pi)/(2))=-1`

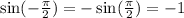
because sine is odd (that is, f(-x) = -f(x)).

next,

`\tan^(-1)(-1)=-\tan^(-1)(1)=-( \pi)/(4)`

again, because the inverse of tangent is odd as well. also, tan(pi/4) is well known to be 1.

finally, we can add the two results. note that 1/2 = 2/4, and we do so to make both denominators the same.

`(1)/(2)-(\pi)/(4)=(2)/(4)-(\pi)/(4)=(2-\pi)/(4)`