Question

Mathematics: If (f(x) = -3x - 5) and (g of f(x) = logâ¡(x)), for all the values of x, what is the value of c? a) -3 b) -5 c) -8 d) 8

Answer 1

The correct value for c is
`\( \mathbf{c = -5} \).`

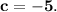
Step-by-step explanation:

In the given problem, we have
`\( f(x) = -3x - 5 \) and \( g(f(x)) = \log_(√(x)) \).`To find the value of c , we substitute f(x)) into g :

`\[ g(f(x)) = \log_(√(x))(-3x - 5) \]`

Now, to determine c , we set the argument of the logarithm equal to 1:

`\[ -3x - 5 = 1 \`

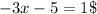
Solving for x , we get:

`\[ -3x = 6 \]\[ x = -2 \]`

Now that we have the value of x , we substitute it back into f(x) to find c :

`\[ f(-2) = -3(-2) - 5 \]\[ f(-2) = 6 - 5 \]\[ f(-2) = 1 \]`

So, c is the value that makes f(x) = 1, which is
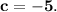`\( \mathbf{c = -5} \).` Therefore, option (b) is the correct answer.

In conclusion, by carefully substituting f(x) into g, setting the logarithmic argument to 1, solving for x , and then finding( c by evaluating f(x) at the determined x,we arrive at the correct answer
`\( \mathbf{c = -5} \)`. This process ensures a systematic and accurate solution to the mathematical problem.