Question

If Sudeep and Michael share sweets in the ratio 15:7 if you then give Michael 36 sweets then the ratio becomes 3:19 how many sweets did they have initially

Answer 1

Initially, Sudeep and Michael had 150 and 70 sweets, respectively.

How to find how many sweets did they have initially

Let's the initial number of sweets that Sudeep and Michael have as 15x and 7x respectively

After giving Michael 36 sweets, his new amount becomes (7x + 36), and the ratio becomes 3:19.

So, we can set up the equation:

`\[(15x)/(7x + 36)` =
`(3)/(19)`

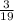
Now, cross-multiplying to solve for x

15x * 19 = 3 * (7x + 36)

285x = 21x + 108

By Subtracting 21x from both sides:

264x = 108

Now, divide by 264 to find x

x =
`(108)/(264)`

Lets multiply by the initial amounts to find the total number of sweets:

15x * 7 + 7x = 15 *
`(108)/(264)` * 7 + 7 *
`(108)/(264)`

`\[15 * (108)/(264) * 7 + 7 * (108)/(264)\]`

`\[ (15 * 108 * 7)/(264) + (7 * 108)/(264)\]`

=
`\[ (1890 + 756)/(264)\]`

=
`\[ (2646)/(264)\]`

= 10

Therefore, initially, Sudeep and Michael had 15 * 10 = 150 and 7 * 10 = 70 sweets, respectively.