Question

A line that includes the points (j, – 3) and (7,6) has a slope of – 9. What is the value of j? j=

Answer 1

j = 8

Explanation:

Slope of a line is given by the formula,

`\boxed{\bf Slope = (y_2-y_1)/(x_2-x_1)}`

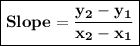
(j, -3) ⇒ x₁= j & y₁ = -3

(7, 6) ⇒ x₂=7 & y₂ = 6

Substitute the values in the above formula,

`\sf (6-(-3))/(7 - j)=-9\\\\\\~~~~~(6+3)/(7-j) = -9\\\\\\~~~~~~(9)/(7-j) = -9`

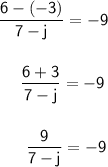
Cross multiply

9 = (-9) *(7 - j)

9 = -9*7 -j *(-9)

9 = -63 + 9j

Add 63 to both sides,

63 + 9 = 9j

72 = 9j

9j = 72

Divide both sides by 9,

j = 72 ÷ 9

`\boxed{\bf j = 8}`