Question

Let (X, d) be a metric space and x0, y0 ∈X. If xn→x0 and yn→y0 prove limn→[infinity]d(xn, yn) = d(x0, y0).

Answer 1

The statement is true. If
`\(x_n \to x_0\)` and
`\(y_n \to y_0\)` in the metric space (X, d), then
`\(\lim_{{n \to \infty}} d(x_n, y_n) = d(x_0, y_0)\).`

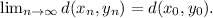
Step-by-step explanation:

In a metric space (X, d), the convergence of sequences is defined with respect to the metric d. If
`\(x_n \to x_0\)` as
`\(n \to \infty\)` and
`\(y_n \to y_0\)` as \(n \to \infty\), it means that the sequences
`\(\{x_n\}\)`
`\(\{y_n\}\)`converge to
`\(x_0\)` and
`\(y_0\)`, respectively. The convergence of sequences in a metric space implies convergence of the metric applied to those sequences. Therefore,
`\(\lim_{{n \to \infty}} d(x_n, y_n) = d(x_0, y_0)\)`, confirming the result.

To provide a brief proof, consider the definition of convergence: for every
`\(\epsilon > 0\)`, there exists N such that for all
`\(n \geq N\), \(d(x_n, x_0) < \epsilon\)` and
`\(d(y_n, y_0) < \epsilon\)`. Then, the triangle inequality for metrics is applied
`\(d(x_n, y_n)\)`, ensuring that
`\(\lim_{{n \to \infty}} d(x_n, y_n) = d(x_0, y_0)\).`

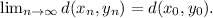
In conclusion, the convergence of sequences
`\(x_n\)` and
`\(y_n\)` in a metric space implies the convergence of the metric
`\(d(x_n, y_n)\)` to
`\(d(x_0, y_0)\)` as
`\(n \to \infty\)`. This property is fundamental in understanding the continuity and convergence concepts in metric spaces.