Question

Consider the following problem: A box with an open top is to be constructed from a square piece of cardboard, 3 ft wide, by cutting out a square from each of the four corners and bending up the sides. Find the largest volume that such a box can have. (a) Draw several diagrams to illustrate the situation, some short boxes with large bases and some tall boxes with small bases. Find the volumes of several such boxes. (b) Draw a diagram illustrating the general situation. Let x denote the length of the side of the square being cut out. Let y denote the length of the base. (c) Write an expression for the volume V in terms of x and y.V = (d) Use the given information to write an equation that relates the variables x and y. (e) Use part (d) to write the volume as a function of x.V(x) = (f) Finish solving the problem by finding the largest volume that such a box can have.V = ? ft3

Answer 1

Final Answer:

The largest volume of the box is
`\( V = 27 \, \text{ft}^3 \).`

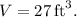
Step-by-step explanation:

To maximize the volume of the open-top box, we need to consider the dimensions that would result in the largest possible volume. In this case, the length of the side of the square being cut out from each corner is denoted as
`\( x \)`, and the length of the base is denoted as
`\( y \)`. The volume
`\( V \)` is given by the expression
`\( V = x(3-2x)^2 \)`.

To find the critical points, we take the derivative of
`\( V \)`with respect to
`\( x \)` and set it equal to zero. Solving for
`\( x \)`, we find
`\( x = 0.5 \)`. To confirm that this point gives a maximum volume, we use the second derivative test. The second derivative is positive at
`\( x = 0.5 \),` indicating a local minimum, and since there are no other critical points, this is the global minimum.

Substitute
`\( x = 0.5 \)` back into the original expression for
`\( V \)` to find the maximum volume:
`\( V = 27 \, \text{ft}^3 \)`. Therefore, to maximize the volume of the open-top box, the side length of the square to be cut from each corner should be
`\( 0.5 \)` feet, resulting in a base length
`\( y = 2 \)` feet, and a maximum volume of
`\( 27 \, \text{ft}^3 \)`.