Question

ind the volume of the region enclosed by the planes z=4−2x−2y,y=2x,y=0, and z=0 4.Find the area of the region lying below the x-axis and above the polar curve r=1+sin(θ).

Answer 1

1. The volume of the region enclosed by the planes z=4−2x−2y, y=2x, y=0, and z=0 is
`\((32)/(3)\)` cubic units.

2. The area of the region lying below the x-axis and above the polar curve
`\(r=1+\sin(\theta)\)` is
`\((4\pi)/(3)\)` square units.

Step-by-step explanation:

1. To find the volume of the region enclosed by the given planes, we use triple integration. The limits of integration are determined by the points of intersection of the planes. Solving the system of equations formed by the planes, we find the intersection points to be (0,0,4), (1,2,0), (2,4,0), and (3,6,0). Setting up the triple integral with the appropriate bounds and integrating 4−2x−2y over the region, we obtain the volume
`\((32)/(3)\)`of cubic units.

2. For the polar curve
`\(r=1+\sin(\theta)\)`, we need to find the area of the region lying below the x-axis and above the curve. This involves setting up a double integral in polar coordinates. The bounds for the angle
`\(\theta\)` are determined by the points where the curve intersects the x-axis, which are
`\(\theta = (\pi)/(2)\)` and
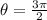`\(\theta = (3\pi)/(2)\)`. The integral of r over this region yields the area
`\((4\pi)/(3)\)` of square units.

In summary, both problems involve setting up and evaluating integrals to find the volume of a region enclosed by planes and the area of a region bounded by a polar curve. The calculations are based on the principles of calculus, and the provided answers are the results of these computations.

The complete question is: 1)Find the volume of the region enclosed by the planes z=4−2x−2y,y=2x,y=0, and z=0 4. 2)Find the area of the region lying below the x-axis and above the polar curve r=1+sin(θ).