Final Answer:
The solution to the given initial value problem is
 .
.
Step-by-step explanation:
To solve the differential equation
 with the initial condition
with the initial condition
 , we can recognize it as a first-order linear differential equation. To solve it, we can use the method of separating variables.
, we can recognize it as a first-order linear differential equation. To solve it, we can use the method of separating variables.
The given equation can be rearranged as
 . Now, separate variables by dividing both sides by
. Now, separate variables by dividing both sides by
 :
:
![\[(1)/(4x + y)dx = -(1)/(x - y)dy\]](https://img.qammunity.org/2024/formulas/mathematics/college/n19y0kpq6qv2ps5cgbj71mejgni660ys6o.png)
Now, integrate both sides separately:
![\[\int (1)/(4x + y)dx = -\int (1)/(x - y)dy\]](https://img.qammunity.org/2024/formulas/mathematics/college/w1exf1wpsig94s3udeqet8hrbjozjp5t1l.png)
This leads to
 , where
, where
 is the constant of integration. Simplifying further, we get:
is the constant of integration. Simplifying further, we get:
![\[\ln|4x + y| + \ln|x - y| = C\]](https://img.qammunity.org/2024/formulas/mathematics/college/44riro3286nppybelhtlgrkp9rykt77cwb.png)
Combine the logarithms using the properties of logarithms:
![\[\ln|4x + y(x)||x - y(x)|| = C\]](https://img.qammunity.org/2024/formulas/mathematics/college/791abhslrwyszw51uhjiirp38c6jp3lmf6.png)
Exponentiate both sides:
![\[|4x + y(x)||x - y(x)| = e^C\]](https://img.qammunity.org/2024/formulas/mathematics/college/1ij4vxe0vaajjdu4fwgg03w91t14bfmbhj.png)
Now, consider the initial condition
 to find the value of
to find the value of
 . Substituting
. Substituting
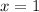 and
and
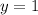 into the equation, we get
into the equation, we get
 , so
, so
 .
.
Substitute
 back into the equation, simplify, and solve for
back into the equation, simplify, and solve for
 to obtain
to obtain
 , which is the solution to the initial value problem.
, which is the solution to the initial value problem.