Question

The equation y =5/9 (x-32) relates a temperature given in x degrees Fahrenheit to the corresponding temperature y measured in degrees Celcius. a. Solve the equation y = 5/9(x - 32) for x to write x (Fahrenheit temperature) in terms of y (Celcius temperature). b. Let C(x) = 5/9(x - 32) be the function that takes a Fahrenheit temperature as input and produces the Celcius temperature as output. In addition, let F(y) be the function that converts a temperature given in y degrees Celcius to the temperature F(y) measured in degrees Fahrenheit. Use your work in (a) to write a formula for F(y). c. Next consider the new function defined by p(x) = F(C(x)). Use the formulas for F and C to detemine an expression for p(x) and simplify this expression as much as possible. What do you observe?

Answer 1

a.
`\(x = (9)/(5)y + 32\)`

b.
`\(F(y) = (9)/(5)y + 32\)`

c.
`\(p(x) = x\)`

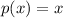
Step-by-step explanation:

a. To solve
`\(y = (5)/(9)(x - 32)\) for \(x\)`, first multiply both sides by
`\((9)/(5)\)` to isolate (x). This gives
`\(x = (9)/(5)y + 32\)`, providing a conversion formula from Celsius to Fahrenheit.

b. Given
`\(C(x) = (5)/(9)(x - 32)\) and \(x = (9)/(5)y + 32\)` from part (a), we need a formula for (F(y)), converting Celsius to Fahrenheit. Substituting the expression for (x) from (a) into (F(y)) gives
`\(F(y) = (9)/(5)y + 32\)`, which represents the Fahrenheit temperature in terms of Celsius.

c. The function (p(x)) is defined as (p(x) = F(C(x))). Substituting (C(x) =
`(5)/(9)(x - 32)\)` into (F(y) =
`(9)/(5)y + 32\)` gives
`\(p(x) = (9)/(5)((5)/(9)(x - 32)) + 32\)`. Simplifying this expression results in (p(x) = x). This reveals that (p(x)) is simply equivalent to the input (x) without any change, demonstrating that converting from Celsius to Fahrenheit and back to Celsius yields the original value.

The observation here is that the composition of functions (F) and (C) to form (p(x)) results in a function that essentially returns the input value (x) without alteration. This occurs due to the nature of the conversion formulas between Celsius and Fahrenheit canceling each other out when applied consecutively.

It emphasizes the symmetry and inverse relationship between the Celsius and Fahrenheit temperature scales, showcasing that converting between the two and then back yields the original temperature value.