Question

F(x)=(1)/(7)x-3 is one inverse of t domain and range

Answer 1

The inverse function of
`\( f(x) = (1)/(7)x - 3 \) is \( f^(-1)(x) = 7x + 21 \)`. Both functions have unrestricted domains, encompassing all real numbers, and their ranges also span the entire set of real numbers. This is due to the linear nature of the functions, ensuring a one-to-one correspondence between inputs and outputs across the real number line.

Step-by-step explanation:

To find the inverse function of
`\( f(x) = (1)/(7)x - 3 \)`, we can interchange x and y and solve for y:

`\[ y = (1)/(7)x - 3 \]`

Interchanging x and y:

`\[ x = (1)/(7)y - 3 \]`

Now, solve for y:

`\[ x + 3 = (1)/(7)y \]`

Multiply both sides by 7 to isolate y:

`\( 7(x + 3) = y \\\( y = 7x + 21 \\`

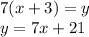
So, the inverse function of
`\( f(x) = (1)/(7)x - 3 \) is \( f^(-1)(x) = 7x + 21 \)`.

Now, let's talk about the domain and range:

For the original function
`\( f(x) = (1)/(7)x - 3 \)`:

The domain is all real numbers because there are no restrictions on x.

The range is also all real numbers because the function is a linear function with a slope of
`\( (1)/(7) \)`, so it covers all possible values of f(x).

For the inverse function
`\( f^(-1)(x) = 7x + 21 \)`:

The domain is all real numbers because there are no restrictions on x.

The range is also all real numbers because the inverse function is a linear function with a slope of 7, covering all possible values of
`\( f^(-1)(x) \)`.

Full Question:

Find the inverse function of
`\( f(x) = (1)/(7)x - 3 \)` and describe the domain and range of both the original function and its inverse.