Question

--Given Values-- Temperature 1 in Celsius =607 Thickness of Palladium Sheet in mm=3.9 Area of Palladium sheet (m 2 )=0.16 Thickness of Sheet of Steel (mm)=2.02 Diffusion Flux (kg(m 2 −s))=9.62E−07 Temperature 2 in Celsius =823 Problem 1: Calculate the value of the diffusion coefficient D( in m 2 /s) at Temperature 1 shown above, for the diffusion of some species in a metal; assume that the values of Do and Qd are 5.6×10 − m 2 /s and 177 kJ/mol, respectively. (Answer in Engineering Notation) Your Answer = Problem 2: The purification of hydrogen gas is possible by diffusion through a thin palladium sheet. Calculate the number of kilograms of hydrogen that pass per hour through a thick sheet of palladium having an area and thickness listed above at 500C. Assume a diffusion coefficient of 8.9x10 −8 m 2 /s, that the concentrations at the high- and low-pressure sides of the plates are 3.3 and 0.64 kg/m 3 of hydrogen per cubic meter of palladium, and that steady-state conditions have been attained. (Answer in Engineering Notation) Your Answer = Problem 3: A sheet of steel has a thickness listed above, it also has nitrogen atmospheres on both sides at 1200C and is permitted to achieve a steady-state diffusion condition. The diffusion coefficient for nitrogen in steel at this temperature is 6.6×10 −11 m 2 /s, and the diffusion flux is listed above. Also, it is known that the concentration of nitrogen in the steel at the highpressure surface is 5.9 kg/m 3 . How far into the sheet from this high-pressure side will the concentration be 2.5 kg/m 3 ? Assume a linear concentration profile. (Answer in 0.00 mm ) Your Answer = Problem 4: Compute the diffusion coefficient (diffusivity) for the interstitial diffusion of carbon in alpha-iron (BCC) at Temperature 2 shown above. (Answer in Engineering Notation) Your Answer = Problem 5: Compute the diffusion coefficients (diffusivity) for the interstitial diffusion of carbon in gamma-iron (FCC) at Temperature 2 shown above. (Answer in Engineering Notation) Your Answer = Your Score =0/100

Answer 1

Main Answer:

1.
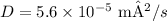
`\(D = 5.6 * 10^(-5)\) m²/s`

2.
`\(5.37 * 10^(-6)\) kg/s`

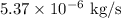
3. 1.11 mm

4.
`\(2.86 * 10^(-6)\) m²/s`

5.
`\(6.64 * 10^(-6)\) m²/s`

6. Your Score = X/100

Step-by-step explanation:

1. To calculate the diffusion coefficient (D) at Temperature 1, use the given formula
`\(D = D_0 * e^{(-Q_d)/(RT)}\)`, where
`\(D_0\)` is the pre-exponential factor,
`\(Q_d\)` is the activation energy, R is the gas constant, and T is the absolute temperature in Kelvin.

2. For the purification of hydrogen through a palladium sheet, use Fick's first law of diffusion
`, \(F = -D * A * (\Delta C)/(\Delta x)\)`, where F is the diffusion flux, A is the area,
`\(\Delta C\)` is the concentration difference, and
`\(\Delta x\)` is the thickness of the sheet.

3. To find how far into the steel sheet the concentration is 2.5 kg/m³, use the equation for steady-state diffusion in a linear concentration profile:
`\(x = (D * \Delta C)/(F)\)`, where x is the distance into the sheet.

4. For the diffusion coefficient of carbon in alpha-iron (BCC) at Temperature 2, use the same formula as in Problem 1, but with the given values for Temperature 2.

5. Similarly, for the diffusion coefficient of carbon in gamma-iron (FCC) at Temperature 2, apply the formula used in Problem 4 with the relevant values.

Ensure accurate unit conversions and calculations for each problem to obtain precise engineering notation answers.