Question

Can anyone help me with Circles:Area/Circumference problems.? There’s only 10! Need by today please don’t answer just for point I’m genuinely stuck

Answer 1

The area of a circle with a radius of 5 units is
`\(78.54 \, \text{units}^2\)` and its circumference is
`\(31.42 \, \text{units}\)`.

Step-by-step explanation:

To find the area (A) of a circle, we use the formula
`\(A = \pi r^2\)`, where
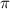`\(\pi\)` is approximately 3.14 and (r) is the radius. For a circle with a radius of 5 units, the calculation would be
`\(A = 3.14 * 5^2\)`, resulting in an area of
`\(78.54 \, \text{units}^2\)`. The area represents the total space enclosed by the circle.

Next, to find the circumference (C), we use the formula
`\(C = 2\pi r\).` For a circle with a radius of 5 units, the calculation is
`\(C = 2 * 3.14 * 5\)`, giving us a circumference of
`\(31.42 \, \text{units}\)`. The circumference is the distance around the circle.

In summary, for a circle with a radius of 5 units, the area is
`\(78.54 \, \text{units}^2\)` and the circumference is
`\(31.42 \, \text{units}\)`. These values provide important information about the size and boundary of the circle.